Reversible Inactivation of α-Amylase at Different Temperatures Volume 53- Issue 1

Christopher J Blowers and Arshad Khan*

• Department of Chemistry, Eberly College of Science, Pennsylvania State University, USA

Received: September 12, 2023;   Published: September 25, 2023

*Corresponding author: Arshad Khan, Department of Chemistry, Eberly College of Science, Pennsylvania State University, DuBois, Pennsylvania 15801, USA

DOI: 10.26717/BJSTR.2023.53.008334

Abstract PDF

A two-step inactivation model of α-amylase was developed by our group in 1993 and 1995 (Lecker & Khan, Biotechnology Progress). In the first step a reversible inactivation takes place followed by an irreversible inactivation step leading to denaturation of the protein. The earlier studies by our group primarily examined the irreversible inactivation (35-50 °C) of the protein that increases with time and finally approaches complete (100%) inactivation. Contrary to that, the present reversible inactivation study shows an increase in inactivation with time (35- 50 °C), reaching a maximum and then decreasing to an equilibrium value. The maximum is most pronounced at a lower temperature (35 °C) and is significantly reduced as the temperature is increased to around 50 °C. The increased reversible inactivation is due to dissociation of Ca²⁺ ions that dominates at the beginning followed by a decreased inactivation due to association of Ca²⁺ ions to apoenzyme.

α-Amylase is a metallo-enzyme with medicinal, therapeutic, and industrial applications [1]. It helps in the digestion of carbohydrates and is secreted by pancreas and salivary glands. This enzyme contains at least 1 mol of calcium ion [2-5] per mole of protein. The strength of the binding of calcium ions to the protein varies significantly from one source of enzyme to another. Irrespective of the source, α-amylase causes a rapid fragmentation of starch molecules into sugars [6-8] and undergoes inactivation reaction initiated by an initial dissociation of Ca²⁺ ions followed by denaturation by heat [9-11]. The theory of inactivation was developed by our group some 30 years ago and confirmed the validity of its predictions by quite a few experiments in aqueous [9,10] as well as non-aqueous solvents [11]. The earlier studies primarily focused on irreversible inactivation, and the effects of temperature and solvent on this inactivation [9-11]. Unfortunately, not much effort was made to study the reversible inactivation and effect of temperature on this step of inactivation [12]. In this paper we first present the theory in brief before describing the temperature effect on reversible inactivation followed by a comparison between reversible and irreversible inactivation.

The first reversible stage (eq 1) involves a forward reaction that forms an inactive apoenzyme, E^2-, from the active enzyme, CaE, with a rate constant of k₁ and a reverse reactivation reaction involving the combination of E^2- with calcium ions with a rate constant of k_-1. The second irreversible stage (eq 2) of reaction forms a denatured form of the enzyme, EI^2-, from E^2- with a rate constant of k₂. The reversibly inactivated form, E^2-, can be quickly transformed into the active form, CaE, by adding calcium ions. On the other hand, the EI^2- is the denatured form of the enzyme that cannot be reactivated by adding calcium ions.

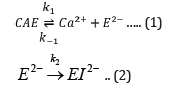
Based on inactivation steps 1 and 2, the following equations are derived:

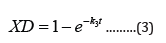
The expression XD (eqn 3) gives the fraction of enzyme inactivated at a time, t, after the inactivation process begins, and k₃, given by equation 4, is a function of calcium ion concentration and temperature. From the expression 3 one can readily obtain the value of percent active enzyme as follows:

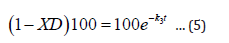
A more detailed derivation of equation 3 (and hence, eqn 5) as well as various test results can be found in references [9,10].

Reversible Inactivation of α-Amylase from Total and Irreversible Inactivation

Based on the model that we put forward earlier [9,10] we can write the following expression:

In other words,

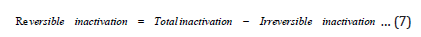
In our earlier paper we described how to determine the total and irreversible inactivation [9] of the protein based on experiments with and without the added calcium chloride (provides calcium ions in the solution). Without the added calcium ions, one gets the total inactivation, and by adding calcium ions one gets the irreversible inactivation as reversible process of reaction 1 is stopped as excess Ca²⁺ ions shift the eqn. 1 equilibrium to the left (Le Chatlier’s principle) resulting in the formation of undissociated metallo-enzyme (CaE). Here, we determine the reversible inactivation values by applying eqn. 7 Although quite a number of studies were performed on irreversible inactivation, to the best of our knowledge the reversible inactivation values and the effect of temperature on these values were not reported earlier. To understand the enzyme inactivation completely, such a study was essential. The importance of these inactivation values has motivated us to work on reversible inactivation.

Temperature Effect on Reversible Inactivation: Comparison with Total and Irreversible Inactivation

The upper most curve (red) of Figure 1 represents the effect of temperature on total inactivation of the enzyme (experiment without the added Ca²⁺ ions) followed by the one (blue) with irreversible inactivation (experiment with added Ca²⁺ ions). The subtracted curve is shown at the bottom and represents the reversible inactivation. All these values represent those obtained at 35 °C. As one would notice that the total inactivation (upper most curve) as well as the irreversible inactivation (second curve from the top) increases with time and approaches 100% inactivation with time. However, the reversible inactivation (bottom curve) rises to a maximum and then decreases to a small constant value with time. A similar trend is also noticed at other temperatures. Figure 2 shows a plot of reversible inactivation values at different temperatures. The upper most curve represents the 35 °C curve (blue) followed by 40 °C curve (red) showing very similar trend except the fact that the peak is very much reduced and shifted to smaller time as temperature is increased from 35 to 40 °C. While the peak at 35 °C appears at around 30 min, the peak at 40 °C appears at around 15 min, and 50 °C (grey curve) at around 5 min.

Figure 1

Figure 2

Explaining the Reversible Inactivation Trend

We can explain our results based on the reversible inactivation step that we put forward earlier [9].

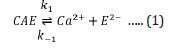
Each reversible inactivation curve (as in Figure 1, bottom curve) shows an increase in inactivation with time before reaching a maximum and then decreasing to a constant value. The increase in inactivation at the beginning is due to dissociation reaction (eqn. 1, forward reaction) in which Ca²⁺ ions separate from the active enzyme, CaE forming a reversibly inactive apoenzyme (E^2-). As the concentration of Ca²⁺ ions increase in the solution the reverse association reaction takes place forming active enzyme, CaE and thus, reducing the fraction of inactive enzyme. After some time, an equilibrium is reached leveling off the inactivation values as seen in Figure 1 bottom curve. As the temperature is increased from 35 °C to 40 and 50 °C, the peak height is reduced and shifted to shorter time when the reverse activation reaction sets in. To further prove our hypothesis of reduced inactivation due to association of Ca²⁺ ions, we presented here results of an EDTA experiment that binds with free calcium in the protein solution. In the absence of free Ca²⁺ ions, the association reaction will be stopped, and the inactivation will not be expected to decrease. Indeed, that is the case for 15 °C curve obtained with EDTA being added to protein solution. Under this situation, all the free calcium ions will be removed by EDTA and the reactivation reaction will not take place at any measurable rate. This is why the green curve in Figure 2 (almost a straight line) does not show any distinct peak as in other curves obtained at 35, 40 and 50 °C.

α-Amylase inactivates in two stages, a reversible inactivation followed by an irreversible inactivation leading to denaturation of the protein. In earlier work we presented how at any temperature the inactivation of the active enzyme takes place and approaches 100% inactivation with time. Contrary to that the reversible inactivation first increases, reaches a maximum and then decreases to an equilibrium value. All these observations are explained by our two-step model.

1. Azzopardi, Catherine Lloyd, Sofia Rodrigues Teixeira, R Steven Conlan, Iain S Whitaker, et al. (2016) Clinical Applications of amylase: Novel perspectives. Surgery 160: 26-37.
2. Kakiuchi K, S Kato, A Imanishi, T Isemura (1964) Association and dissociation of Bacillus subtilis ɑ-amylase. J Biochem 55: 102-109.
3. Whitaker J R (1972) In Principles of Enzymology for the Food Sciences; New York: Dekker, pp. 433-467.
4. Boel E (1990) Calcium binding in a-amylases: An X-ray diffraction study at 2.1 -Å resolution of two enzymes from Aspergillus. Biochemistry 29: 6244-6249.
5. Chandel AK, Rudravaram R, Rao LV, Pogaku R, Narasu ML, et al. (2007) Industrial enzymes in bioindustrial sector development: An Indian perspective. J Comm Biotechnol 13: 283-291.
6. Manners DJ (1962) Enzymic synthesis and degradation of starch and glycogen. Adv Carbohydr Chem 17: 371-430.
7. Thoma JA, Spradlin JE, Dygert S, Boyer P D (1971) The Enzymes 5: 115-189.
8. Takagi T, Toda H, Isemura T (1971) Bacterial and mold amylases. The Enzymes 5: 235-271.
9. Lecker DN, Khan A (1996) Theoretical and experimental studies of the effects of heat, EDTA, and enzyme concentration on the inactivation rate of α –amylase from Bacillus sp. Biotechnol Prog 12: 713-717.
10. Lecker DN, Khan A (1998) Model for inactivation of α -amylase in the presence of salts: theoretical and experimental Studies. Biotechnol Prog 14: 621-625.
11. Calabrese VT, Minns JW, Khan A (2016) Suppression of α-Amylase inactivation in the presence of Ethanol: Application of a two-step model, Biotechnology Progress 32(5): 1271-1275.
12. Morlyoshi T, Ishll T, Tamal Y, Tado M (1990) Static Dielectric Constants of Water + Ethanol and Water + 2-Methyl-2-propanol Mixtures from 0.1 to 300 MPa at 298.15 K. J Chem Eng Data 35(1): 17-20.